Gas compression is a fundamental process employed in various industries, including oil and gas, manufacturing, and energy production. It involves increasing the pressure of a gas, making it an essential part of many industrial processes.
It is done to increase the pressure of the gas, this is accompanied by change of state of the gas which means change in temperature and volume of a quantum of gas going under compression.
If the pressure of gas is raised from P1 to P2, the compression ratio for this process is defined as, P1 / P2. The physical state of gas will undergo change depending on the process, whether it is isothermal, adiabatic or typically polytropic.
In this post, we will delve into the principles of gas compression, its applications, and the equipment used in this vital process.
INDEX
Principles Of Gas Compression
Boyle's Law
Charles's Law
Adiabatic Compression
Isothermal Compression
Polytropic Compression
Applications Of Gas Compression
Gas Compression Equipment
Principles Of Gas Compression
Gas compression reduces the volume of a gas while simultaneously increasing its pressure. The primary objective is to store, transport, or facilitate the downstream processing of the gas. There are several principles that underlie gas compression:
Boyle's Law
This fundamental principle states that for a given amount of gas at constant temperature, the pressure and volume are inversely proportional. When the volume decreases, the pressure increases, and vice versa. Gas compression capitalizes on this principle to increase the pressure of the gas.
In mathematical terms, Boyle's Law can be expressed as:
P₁V₁ = P₂V₂
Where:
-
- P₁ is the initial pressure of the gas.
- V₁ is the initial volume of the gas.
- P₂ is the final pressure of the gas (after a change in volume).
- V₂ is the final volume of the gas (after a change in pressure).
Charles's Law
This law describes the relationship between temperature and volume, stating that, at constant pressure, the volume of gas is directly proportional to its absolute temperature. Gas compressors must consider the potential temperature changes during the process. In mathematical terms, Charles's Law can be expressed as:
V₁/T₁ = V₂/T₂
Where:
-
- V₁ is the initial volume of the gas.
- T₁ is the initial absolute temperature of the gas (measured in Kelvin).
- V₂ is the final volume of the gas (after a change in temperature).
- T₂ is the final absolute temperature of the gas (measured in Kelvin).
Adiabatic Compression
In adiabatic compression, there is no heat exchange with the surroundings. This is a common scenario in many compression systems. The compression work done on the gas raises its temperature, and if not managed properly, this can lead to overheating.
For gas compressors without any cooling, gas temperature rises with rise in pressure. The temperature rise is given by the equation,
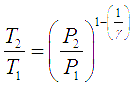Here the subscripts 1 and 2 correspond to initial and final states of the gas respectively. 
is the ratio of specific heat at constant pressure to specific heat for constant volume for a gas (Cp/Cv). For such an adiabatic process, the head developed and power consumed by the compressor are given by following two equations.
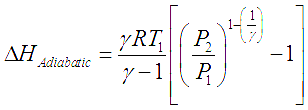This developed pressure head when multiplied by the inlet volumetric flowrate of the gas (V) gives shaft power required to drive an ideal adiabatic compressor, given by following equation,
In these equations R is the ‘Gas constant’ which is obtained by dividing the ‘Universal gas constant’ by molecular weight of a particular gas.
Isothermal Compression
When the gas being compressed in a compressor is cooled with jacketed flow of a coolant, the process is an isothermal process. The work done in this case and hence the power required to run this type of cooled compressors is the theoretically minimum possible limit. This minimum possible power to compress a gas from P1 to P2 is given by following equation,
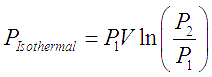Here subscripts 1 and 2 correspond to inlet and outlet states of the gas, V is the inlet volumetric flow of gas.
Polytropic Compression
Adiabatic (constant entropy) and isothermal (constant temperature) compression processes are ideal and theoretical in nature. The actual compression processes are polytropic and typically described with the equation,
Note that when n is replaced by , this process becomes adiabatic. If, n > the work done for polytropic compression and power required to run such a compressor is more than that required for and ideal frictionless adiabatic compressor. We get the head developed and power required for polytropic gas compression by replacing by n in the equations for adiabatic gas compression.
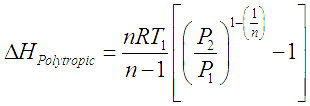
In these equations R is the ‘Gas constant’ which is obtained by dividing the ‘Universal gas constant’ by molecular weight of a particular gas. V is inlet volumetric flow of gas.
Applications Of Gas Compression
Gas compression finds diverse applications across various industries, including:
- Oil and Gas Industry: Compression is critical in the transportation of natural gas through pipelines. It helps maintain the pressure required for efficient flow and delivery to end-users.
- Manufacturing: Compressed air is widely used in manufacturing for pneumatic tools, conveyor systems, and even HVAC systems. It provides a source of reliable power for a range of applications.
- Energy Production: Gas turbines in power plants require compressed air for combustion. Additionally, compressed air is used to operate various control systems in power generation facilities.
- Chemical and Petrochemical Industry: Many chemical processes rely on compressed gases, whether it's for mixing, transportation, or chemical reactions.
- Environmental Control: In wastewater treatment plants, gas compression is used for aeration, and in refrigeration systems, it is essential for the cooling process.
Gas Compression Equipment
To perform gas compression efficiently, various types of equipment are employed:
- Compressors: These are the primary machines used to compress gases. The most common types include reciprocating, centrifugal, and rotary compressors. Each has its own advantages and is chosen based on the specific requirements of the application.
- Cooling Systems: To manage the increase in temperature during compression, cooling systems are incorporated. This can involve intercoolers, aftercoolers, or even refrigeration systems, depending on the situation.
- Filters and Separators: Gas compressors typically include filters and separators to remove impurities, such as moisture and particulates, from the gas stream.
- Control and Monitoring Systems: Compression processes require sophisticated control systems to maintain optimal operating conditions and ensure safety. These systems also monitor for any deviations from the desired parameters.
- Piping and Valves: The transport of compressed gas often necessitates a network of piping and valves to direct the flow and control the pressure.
